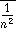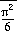One of the well-known series that comes up now and then is the sum of 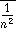; I think it was Euler who showed that this sum is .

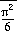Here's a proof that I heard of long ago, due, I think, to Zagier. Let

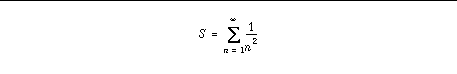
By breaking the sum into even and odd terms, we get
hence we get

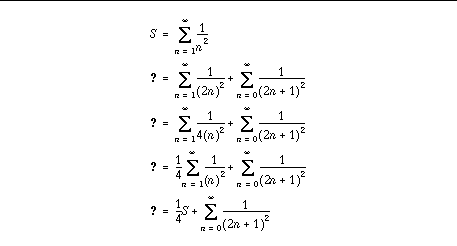
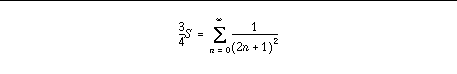
Minor digression: recall from calculus that

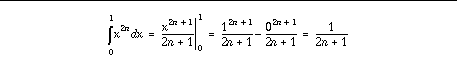
So we can replace the individual terms in the sum to get

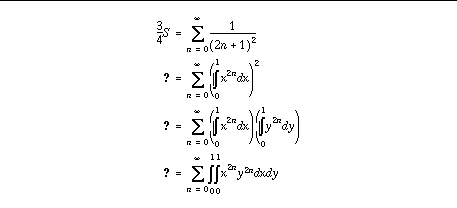
Now we use "engineer's prerogative" to pull the summation inside the integral and get

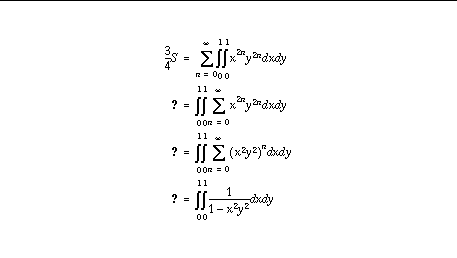
(The last step follows from the formula for the sum of a geometric series.) Now this may not seem like a terribly simple integral, but a remarkable substitution, namely

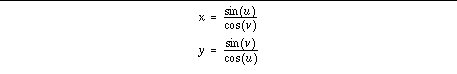
leads to an almost unbelievable Jacobian for the change of variables formula; the simplification goes like this:

Now this means that

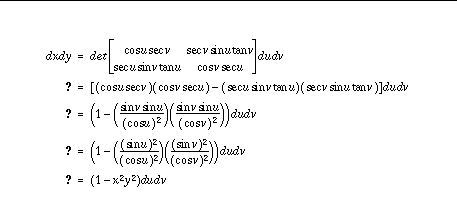
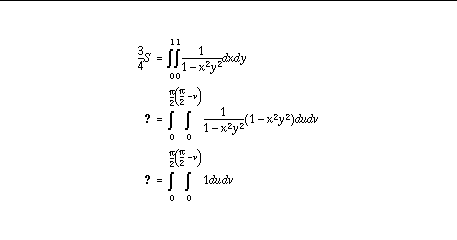
Well, the integral of 1 over a region is the area of that region, which in this case is a 45-45-90 triangle whose base is 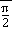, so its area is 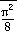. Hence 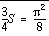, so .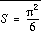

The two real mysteries are (1) why does the change of variables map the unit square to the triangle described above, and (2) how would anyone ever think of all these stunts?

My conjecture for item 2 is that this is really just the unravelling of the standard Fourier series proof (although I've yet to verify this). For item 1, I have to confess that I had Mathematica plot the thing for me and became convinced, although I'd never have thought of it myself.